Eddy Current Loss: Important Applications & Expression

Eddy current loss, also known as Foucault or joule loss, is a phenomenon that occurs when a conductor is exposed to a varying magnetic field, resulting in the generation of circulating currents within the conductor. These circulating currents are known as Eddy currents and are responsible for the dissipation of energy in the form of heat. Eddy current loss can have significant implications in various electrical and magnetic systems.

When a conductor is subjected to a changing magnetic field, such as when it moves through a magnetic field or when the magnetic field itself changes, a voltage is induced across the conductor due to Faraday’s law of electromagnetic induction. According to Lenz’s law, the direction of the induced current opposes the change in the magnetic field that produced it. As a result, the induced current circulates within the conductor, creating closed loops known as eddy currents.

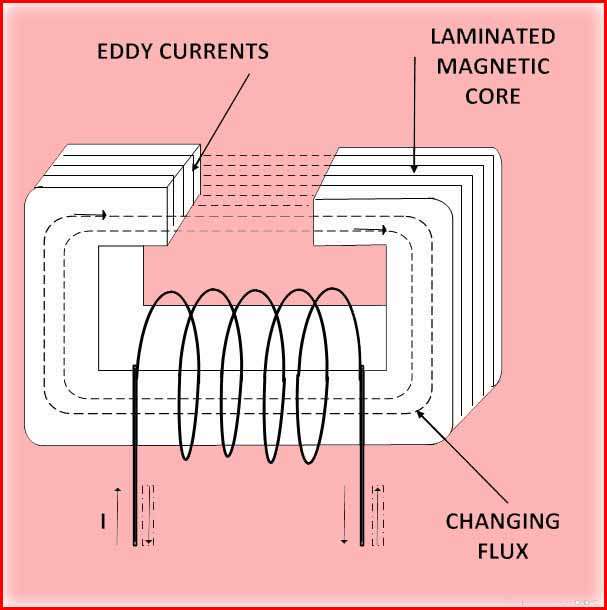
Eddy currents tend to concentrate near the surface of the conductor, particularly in materials with high electrical conductivity, such as copper or aluminum. This is due to the skin effect, which causes the current to flow primarily in a thin surface layer of the conductor, minimizing the resistance and maximizing the eddy current magnitude.

Eddy currents have several effects, the most notable of which is the conversion of electrical energy into heat. As the eddy currents flow through the conductor, they encounter resistance, leading to Joule heating. This dissipated heat represents a loss of energy and can be detrimental in various applications where energy efficiency is crucial, such as electrical machines, transformers, and magnetic braking systems.

The magnitude of eddy current loss depends on several factors, including the strength and frequency of the magnetic field, the electrical conductivity and thickness of the conductor, and the shape and size of the conductor. Higher magnetic field strength or frequency, larger conductor surface area, and higher electrical conductivity increase the eddy current magnitude, resulting in more significant eddy current losses.

To mitigate eddy current losses, several techniques are employed. One common approach is to use laminated cores in transformers and electrical machines such as shown in below figure.

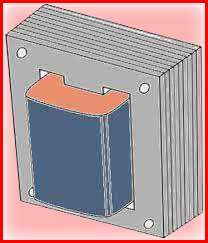
Laminated cores consist of thin layers of conductive material, typically steel, insulated from each other. By introducing insulation between the layers, the eddy currents are confined within individual layers, reducing their magnitude and consequently minimizing the associated losses.

In some cases, magnetic materials with high electrical resistivity, such as ferrites, are used to reduce eddy current losses. These materials exhibit lower electrical conductivity compared to metals, which limits the magnitude of eddy currents and lowers the associated power dissipation.

Mathematical expression for eddy current loss

The mathematical expression for calculating eddy current loss is derived from the power dissipated by the eddy currents in a conductor. The power dissipation, P, can be determined using the following formula:

P = Ke * B^2 * f^2 * t^2 * V^2

Where:

Ke is the eddy current coefficient, which depends on the material properties and geometry of the conductor.

• B is the magnetic field strength.
• f is the frequency of the magnetic field.
• t is the thickness of the conductor.
• V is the volume of the conductor.

The eddy current coefficient, Ke, is a material-specific constant and is given by:

Ke = (π^2 * ρ) / (6 * σ)

Where:

• π is the mathematical constant pi (approximately 3.14159).
• ρ is the resistivity of the material.
• σ is the conductivity of the material.

The resistivity, ρ, and conductivity, σ, are material properties that define how well a material conducts electricity.

It’s important to note that the above formula assumes a simple, homogeneous conductor with uniform magnetic field and frequency distribution. In practical scenarios, the calculation of eddy current loss can become more complex, considering factors such as the shape and size of the conductor, magnetic field distribution, and the presence of laminations or other material variations.

Applications of Eddy Current Loss

Eddy current loss has both practical and detrimental applications in various fields. Let’s explore some of the key applications of eddy current loss:

Heating Applications: Eddy current loss is utilized in induction heating systems. Induction cooktops and industrial heating processes employ high-frequency alternating magnetic fields to generate eddy currents in conductive materials. These currents produce localized heating, enabling efficient and precise heating of the desired area without the need for direct contact or heating elements.

Magnetic Braking and Damping: Eddy current loss is employed in electromagnetic brakes and dampers. When a conductor moves through a magnetic field, the resulting eddy currents create resistance, generating a braking force or damping effect. This mechanism is used in applications such as roller coasters, trains, and damping systems for vibration reduction.

Eddy Current Testing: Eddy current loss is utilized in non-destructive testing (NDT) techniques to assess the integrity of conductive materials. By inducing eddy currents in the material under inspection, flaws, cracks, or defects can be detected. Eddy current testing is commonly used in industries such as aerospace, automotive, and manufacturing to inspect metal components for flaws without causing damage.

Eddy Current Brakes: Eddy current loss is harnessed in braking systems, particularly in high-speed trains and roller coasters. In these applications, strong magnets induce eddy currents in a metal plate or disc, creating resistance and generating a braking force. Eddy current brakes offer several advantages, including smooth and consistent braking, low maintenance, and absence of frictional wear.

Magnetic Shielding: Eddy current loss plays a role in magnetic shielding applications. In electronic devices or sensitive equipment, unwanted magnetic fields can interfere with proper functioning. Shielding materials with high electrical conductivity, such as mu-metal or conductive foils, are used to redirect and absorb magnetic fields by inducing eddy currents, thereby reducing electromagnetic interference.

Energy Loss Reduction: While eddy current loss is generally undesirable due to energy dissipation, efforts are made to minimize its impact in electrical devices. Laminated cores in transformers and electrical machines, as mentioned earlier, are specifically designed to reduce eddy current losses. By introducing insulation between layers, the eddy currents are confined within individual layers, minimizing power dissipation and improving overall efficiency.

These applications highlight how eddy current loss can be harnessed or mitigated depending on the specific requirements of a system or process. By understanding and effectively managing eddy currents, engineers and scientists can optimize the performance, efficiency, and safety of a wide range of technologies.

More Related Posts:

• What is Hysteresis loss?
• Transformers: Important Types, Features
• Transformer Oil Testing: 9 Important Tests
• 10 Transformer Tests Before Commissioning

Subscribe to our Newsletter “Electrical Insights Daily” to get the latest updates in Electrical Engineering. You can also Follow us LinkedIn to see our latest posts.