Power Triangle: Best Applications You Need to Know
Table of Contents
Introduction
The power triangle is a graphical representation that helps analyze the relationships between real power, reactive power, and apparent power in an electrical circuit. It provides a visual tool for understanding and calculating the power components involved.
The power triangle is a geometric representation used in electrical engineering to analyze power in AC (alternating current) circuits. It involves three key components: real power (P), reactive power (Q), and apparent power (S). Real power (P) represents the actual power consumed or transferred by a circuit and is measured in watts (W).
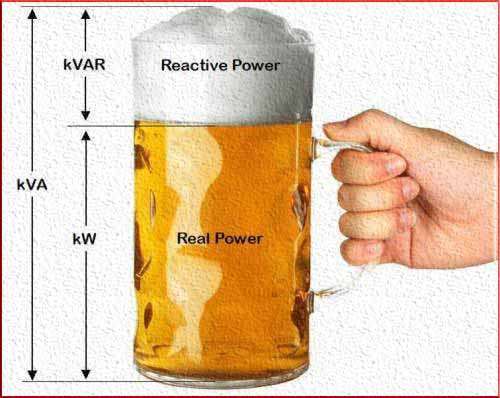
Reactive power (Q) represents the power exchanged between inductive and capacitive elements in a circuit and is measured in volt-amperes reactive (VAR). Apparent power (S) represents the total power flowing in a circuit, including both real and reactive power, and is measured in volt-amperes (VA).
Importance in Electrical Power Analysis
The power triangle is essential for analyzing and understanding power characteristics in electrical systems. It enables the evaluation of power factor, which indicates the efficiency of power transfer in a circuit. The power triangle helps identify power losses, reactive power requirements, and potential issues in electrical networks.
It plays a significant role in power factor correction, load balancing, and optimizing energy consumption in industrial and commercial settings. Understanding the power triangle is crucial for ensuring efficient and reliable power delivery, reducing electricity costs, and improving system performance.
Graphical Representation
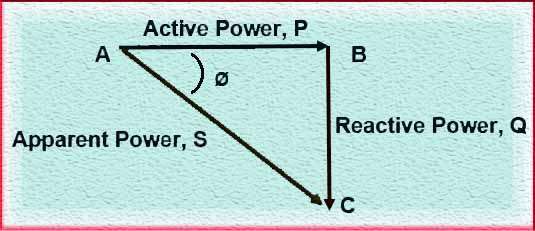
The power triangle is represented as a right triangle. The horizontal base of the triangle represents the real power (P). The vertical side of the triangle represents the reactive power (Q). The hypotenuse of the triangle represents the apparent power (S). The power triangle visually illustrates the magnitudes and relationships between these power components, allowing for a clear understanding of their interdependencies.

The power triangle provides a graphical representation that aids in analyzing and calculating real power, reactive power, and apparent power in electrical circuits. It is a valuable tool for electrical engineers and technicians to assess power characteristics, optimize system efficiency, and ensure reliable power delivery.
Components of the Power Triangle
A. Real Power (P):
Real power (P), also known as active power or true power, represents the actual power consumed or transferred by a circuit. It is the power that performs useful work, such as powering motors, lights, or other electrical devices. Real power is measured in watts (W).
Calculation and Relationship:
Real power (P) is the product of the voltage (V) and current (I) in an AC circuit, multiplied by the power factor (PF).
P = V × I × PF
Real power determines the energy consumption and is the component responsible for generating heat, light, or mechanical work in a circuit.
B. Reactive Power (Q):
Reactive power (Q) represents the power exchanged between inductive and capacitive elements in a circuit. It arises due to the phase difference between the voltage and current waveforms in AC circuits. This power depends on the load nature either its inductive or capacitive. It is measured in volt-amperes reactive (VAR).
Relationship:
Reactive power (Q) is the product of the voltage (V) and current (I) in an AC circuit, multiplied by the sine of the phase angle (θ) between them.
Q = V × I × sin(θ)
Reactive power does not perform useful work but rather oscillates back and forth between the source and reactive components in the circuit.
C. Apparent Power (S):
Apparent power (S) represents the total power flowing in a circuit, including both real power and reactive power. It is the vector sum of real power and reactive power. Apparent power is measured in volt-amperes (VA).
Relationship
Apparent power (S) is the product of the voltage (V) and current (I) in an AC circuit.
S = V × I
Apparent power represents the magnitude of the complex power in a circuit and is the combination of real power and reactive power. Understanding the components of the power triangle, namely real power, reactive power, and apparent power, is essential for analyzing power distribution, optimizing power factor, and ensuring efficient energy usage in electrical systems.
Geometric Representation of the Power Triangle
The power triangle is a graphical representation that visually depicts the relationship between real power, reactive power, and apparent power in an electrical circuit. It is formed as a right triangle, with the real power (P) as the horizontal base, the reactive power (Q) as the vertical side, and the apparent power (S) as the hypotenuse. The power triangle is constructed based on the magnitudes of these power components.
A right triangle has one angle measuring 90 degrees (a right angle). In the power triangle, the right angle is formed between the horizontal base (representing real power) and the vertical side (representing reactive power). The hypotenuse, representing apparent power, is the side opposite the right angle and connects the other two sides of the triangle.
Base, Side, and Hypotenuse Interpretation
The horizontal base of the power triangle represents the real power (P), which is measured in watts (W). The vertical side of the triangle represents the reactive power (Q), which is measured in volt-amperes reactive (VAR).
The hypotenuse of the triangle represents the apparent power (S), which is measured in volt-amperes (VA). The length of each side of the triangle corresponds to the magnitude of the respective power component.
The geometric representation of the power triangle allows for a visual understanding of the relationships and magnitudes of real power, reactive power, and apparent power in an electrical circuit. By examining the triangle, one can observe the relative sizes of the power components and analyze their interdependencies.
Relationship among Power Triangle Components
The power triangle is based on the application of the Pythagorean theorem to the three sides of the right triangle formed by real power (P), reactive power (Q), and apparent power (S).
The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (S) is equal to the sum of the squares of the lengths of the other two sides (P and Q).
Mathematically, this relationship can be expressed as: S^2 = P^2 + Q^2.
Power Factor Angle (θ)
The power factor angle (θ) is the angle between the real power (P) and the apparent power (S) in the power triangle. It represents the phase difference between the voltage and current in an AC circuit. The power factor angle is typically denoted as the angle between 0° and 90°.
The tangent of the power factor angle is equal to the ratio of reactive power (Q) to real power (P). Mathematically, this relationship can be expressed as: tan(θ) = Q / P.
The power factor angle and the power components (P and Q) are interrelated and provide insights into the power characteristics and efficiency of the circuit.
Power Factor (PF)
The power factor (PF) represents the ratio of real power (P) to apparent power (S) in the power triangle. It indicates the efficiency of power transfer in an electrical circuit. Mathematically, the power factor can be calculated as:
PF = P / S.
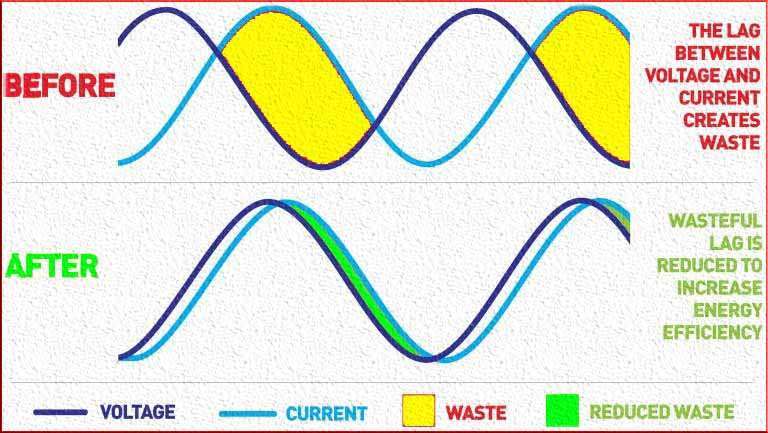
A high power factor (close to 1) indicates efficient power utilization, where real power is a significant portion of the apparent power. A low power factor (close to 0) indicates poor power utilization, where a significant amount of apparent power is reactive power. Improving the power factor through power factor correction techniques can enhance energy efficiency and reduce power losses in electrical systems.
Understanding the relationships among the power triangle components, the Pythagorean theorem, power factor angle, and power factor is essential for analyzing power distribution, evaluating circuit efficiency, and implementing power factor correction measures to optimize energy consumption and improve system performance.
Applications of the Power Triangle
Improving Efficiency and Reducing Losses:
The power triangle is crucial in power factor correction, which aims to improve the power factor of electrical systems. By analyzing the power triangle, engineers can identify circuits with low power factors and implement measures to optimize power factor.
Power factor correction reduces reactive power, minimizes energy losses, and improves the efficiency of power distribution systems.
Methods and Devices for Power Factor Correction:
Capacitors: Adding capacitors to a circuit can offset reactive power, increase power factor, and reduce electricity costs.
Static VAR compensators (SVCs): SVCs are electronic devices that provide reactive power compensation, enhancing power factor and voltage stability.
Synchronous condensers: These rotating machines provide dynamic reactive power compensation and help regulate power factor in large-scale electrical systems.
Analysis of Electrical Systems:
The power triangle allows for the calculation of unknown power quantities in electrical circuits.
Given two known power components (e.g., real power and apparent power), the power triangle can be used to determine the third component (e.g., reactive power).
This is particularly useful in troubleshooting power quality issues, load balancing, and equipment sizing.
Evaluating Power Quality and System Performance:
The power triangle aids in assessing power quality parameters such as power factor, harmonics, and voltage/current distortions.
It helps identify power inefficiencies, voltage drops, and the impact of reactive power on system performance.
Analysis of the power triangle assists in optimizing power usage, reducing energy costs, and improving the reliability of electrical systems.
The applications of the power triangle extend to various industries, including manufacturing, utilities, commercial buildings, and renewable energy systems. By utilizing the power triangle, engineers and technicians can optimize power factor, ensure efficient energy usage, minimize losses, and maintain reliable power delivery in electrical systems.
Energy Cost Reduction:
Power factor correction helps in reducing electricity costs by avoiding penalties imposed by utility companies for low power factors. It reduces reactive power consumption, leading to lower energy bills and improved financial performance.
Power factor correction enables optimal utilization of electrical equipment, such as transformers, motors, and generators, by reducing the reactive power demand.
It allows for better capacity planning and reduces the need for oversized equipment, resulting in cost savings.
Load Balancing:
The power triangle helps identify imbalances in reactive power among different loads in an electrical system. By analyzing the power triangle, engineers can redistribute loads or implement corrective measures to balance reactive power demand.
Load balancing improves the overall system performance, reduces equipment stress, and prevents power quality issues.
Minimizing Voltage Drops
Uneven reactive power distribution can cause voltage drops in electrical systems. By balancing the reactive power, engineers can minimize voltage drops, improve voltage stability, and ensure reliable power delivery.
Power System Analysis
The power triangle assists in analyzing power flow in electrical networks. It allows for the calculation of unknown power quantities, such as real power, reactive power, or apparent power, based on the given information.
Power flow analysis helps in assessing the loading conditions, voltage profiles, and losses in power transmission and distribution systems.
Power Quality Analysis
The power triangle aids in evaluating power quality parameters, such as power factor, harmonics, and voltage/current distortions. It helps identify power inefficiencies, voltage drops, and the impact of reactive power on system performance.
Power quality analysis allows for the identification and mitigation of power quality issues, ensuring reliable and stable power supply.
The applications of the power triangle are crucial in various industries, including manufacturing, utilities, commercial buildings, and renewable energy systems. By utilizing the power triangle, engineers and technicians can optimize power factor, ensure efficient energy usage, minimize losses, and maintain reliable power delivery in electrical systems. It enables cost savings, equipment capacity optimization, load balancing, and effective power system analysis.
Conclusion
In conclusion, the power triangle is a fundamental concept in electrical power analysis and is widely used by engineers and technicians to understand and analyze the relationships between real power, reactive power, and apparent power in electrical circuits.
By representing these power components geometrically, the power triangle provides a visual representation that facilitates analysis and calculations. It helps in determining unknown power quantities, evaluating power factor, and assessing power quality parameters.
The power triangle has practical applications in various areas. Power factor correction is a significant application where the power triangle is used to optimize power factor, improve energy efficiency, and reduce costs. Load balancing, another application, involves redistributing reactive power among different loads to improve system performance and voltage stability. Power system analysis utilizes the power triangle to analyze power flow, assess loading conditions, and evaluate power quality.
Understanding the power triangle and its components allows for efficient energy utilization, reduces power losses, optimizes equipment capacity, and ensures reliable power delivery in electrical systems. It is an essential tool in electrical engineering and plays a crucial role in optimizing power distribution, improving energy efficiency, and maintaining stable power supply.
In conclusion, the power triangle is a valuable tool that enables engineers and technicians to make informed decisions, optimize system performance, and ensure efficient energy utilization in electrical power analysis.
Frequently Asked Questions
What is the formula for the power triangle?
The formula for the power triangle is: Apparent Power (VA) = Real Power (W) + Reactive Power (VAR).
What is the application of power triangle?
The power triangle is commonly used in electrical engineering to visualize and analyze the relationship between real power, reactive power, and apparent power in AC circuits.
What is power triangle in network analysis?
In network analysis, the power triangle represents the geometric relationship between the real power, reactive power, and apparent power in an AC electrical system.
What is the relation between impedance triangle and power triangle?
The impedance triangle represents the relationship between resistance, reactance, and impedance in an AC circuit, while the power triangle shows the relationship between real power, reactive power, and apparent power. Although they involve different quantities, both triangles are used to analyze AC circuits.
What is the full formula of power?
The full formula for power depends on the specific context. In general, power (P) can be calculated using the formula: Power = Voltage (V) × Current (I). However, the formula for power can vary in different scenarios, such as in AC circuits or when considering reactive power.
What is power write formula?
The formula for power is typically written as: P = VI, where P represents power, V represents voltage, and I represents current.
How many types of power are there?
There are generally three types of power in electrical systems: real power (measured in watts, W), reactive power (measured in volt-amperes reactive, VAR), and apparent power (measured in volt-amperes, VA).
What is the significance of power factor?
Power factor is a measure of the efficiency of power usage in an electrical system. It indicates the ratio of real power to apparent power and affects the overall system efficiency, electrical losses, and voltage stability.
What is kVA to kW?
kVA (kilovolt-ampere) represents the apparent power in an electrical system, while kW (kilowatt) represents the real power. The conversion from kVA to kW depends on the power factor of the system. To convert, multiply kVA by the power factor to obtain kW.
What is triangle in logic?
In logic, a triangle is not a specific concept. Logic primarily deals with propositions, truth values, logical operations, and reasoning principles, rather than graphical shapes like triangles.
Is a power triangle a phasor diagram?
The power triangle and phasor diagram are related but distinct concepts. The power triangle represents the relationship between real power, reactive power, and apparent power, while a phasor diagram is a graphical representation of sinusoidal waveforms and their phase relationships in an AC circuit.
What is a kVAR?
kVAR (kilovolt-ampere reactive) represents reactive power in an electrical system. It refers to the power component that oscillates between sources and loads in an AC circuit without performing useful work. Reactive power is measured in VAR (volt-amperes reactive) or kVAR.
What is the difference between kVA and kW?
kVA (kilovolt-ampere) represents the total apparent power in an electrical system, including both real and reactive power components. kW (kilowatt), on the other hand, represents only the real power, which is the actual power used to perform work in the system.
What causes negative kVAR?
Negative kVAR occurs when the reactive power in an electrical system is being supplied rather than consumed. This can happen when capacitive loads, such as power factor correction capacitors, generate reactive power that offsets the reactive power consumed by inductive loads.
Worth Read Posts
Follow us on LinkedIn”Electrical Insights” to get the latest updates in Electrical Engineering. You can also Follow us on LinkedIn and Facebook to see our latest posts on Electrical Engineering Topics.
